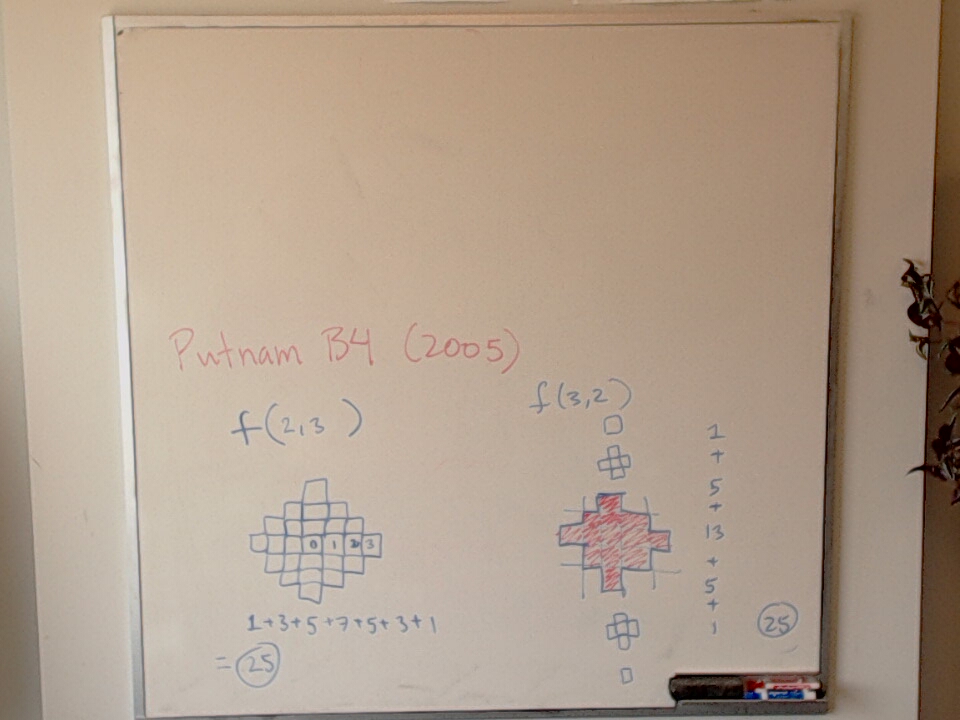
For positive integers $m$ and $n$, let $f(m, n)$ denote the number of $n$-tuples $(x_1, x_2, \dots , x_n)$ of integers such that $|x_1|+|x_2|+ \cdots +|x_n| \leq m$. Show that $f(m, n) = f(n, m)$.
Home / Now / Blog / Notes / Reading / Office Camera / Tags / RSS Feeds / Top of Page
Thanks for reading! If you have any comments or questions about the content, please let me know. Anyone can contact me by email.